Category: Statistical and sparse image models

Line Artefacts Quantification for Lung Ultrasound Images via Non-convex Regularisation

Posted on by o.karakus

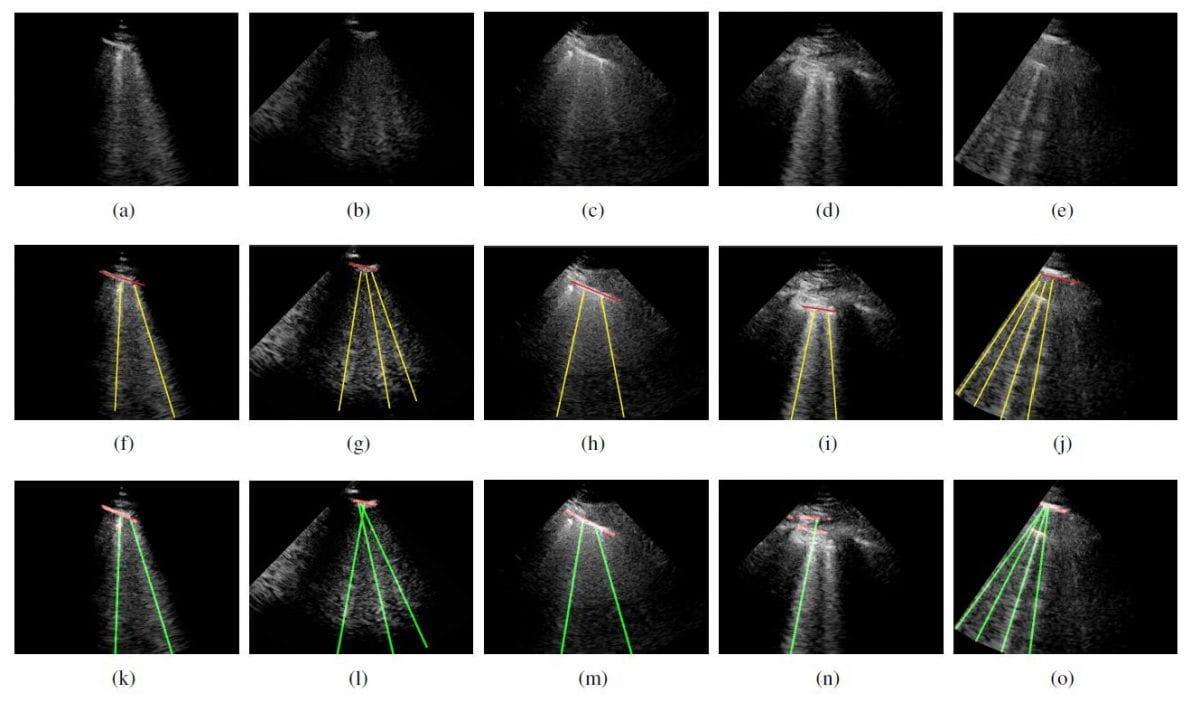
In this project, we present a novel method for line artefacts quantification in lung ultrasound (LUS) images of COVID-19 patients. We formulate this as a non-convex regularisation problem involving the heavy-tailed Cauchy-based penalty function, and the inverse Radon transform.

We employ a simple local maxima detection technique in the Radon transform domain, associated with known clinical definitions of line artefacts.

Line Artefacts in LUS Images
Line artefacts in Radon Domain

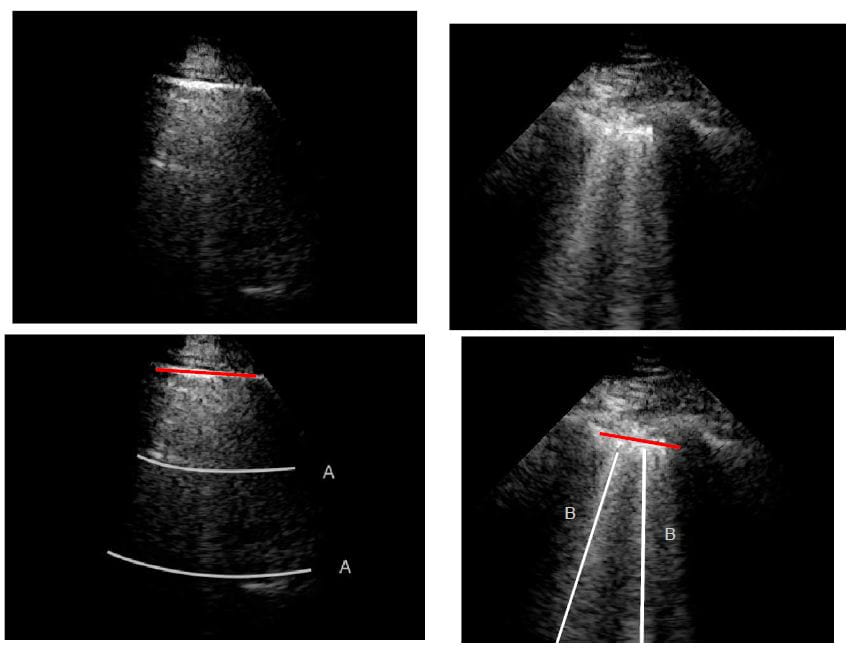
Despite being non-convex, the proposed technique is guaranteed to convergence through our proposed Cauchy proximal splitting (CPS) method, and accurately identifies both horizontal and vertical line artefacts in LUS images. In order to reduce the number of false and missed detection, our method includes a two-stage validation mechanism, which is performed in both Radon and image domains.

We evaluate the performance of the proposed method in comparison to the current state-of-the-art B-line identification method and show a considerable performance gain with 87% correctly detected B-lines in LUS images of nine COVID-19 patients.

Detection results. (a)-(e) Original Images. (f)-(j) Ground truth. (k)-(o) The proposed method.

Further information

[1] Karakuş, O., Anantrasirichai, N., Aguersif, A., Silva, S., Basarab, A., & Achim, A. (2020). Detection of Line Artifacts in Lung Ultrasound Images of COVID-19 Patients Via Nonconvex Regularization. IEEE Transactions on Ultrasonics, Ferroelectrics, and Frequency Control, 67(11), 2218-2229.

Talk

Talk by Dr Oktay Karakus in Acoustics Virtually Everywhere The 179th Meeting of the Acoustical Society of America 7-11 December 2020

https://player.vimeo.com/video/479873161

Contact

Oktay Karakuş, Pui Anantrasirichai, Alin Achim

Code

O Karakus, A Achim. (2020): QuantLUS – CPS v1.0 https://doi.org/10.5523/bris.z47pfkwqivfj2d0qhyq7v3u1i

Funding

This work was supported in part by
** the UK Engineering and Physical Sciences Research Council (EPSRC) under grant EP/R009260/1,
** an EPSRC Impact Acceleration Award (IAA) from the University of Bristol, ** a Leverhulme Trust Research Fellowship to Achim (INFHER).

Ship Wake Detection in SAR Images of the Sea Surface

Posted on by o.karakus
Example images including ship wakes and their Radon transform. (Upper Left) – Image includes all 5 ship wakes. (Upper Right) – Image does not include Kelvin wake. (Lower row) – Radon transforms of the images above. Both images are TerraSAR-X Stripmap products with HH polarisation and 3m by 3m resolution.

Ship wake detection is essential for extracting information on the wake generating vessels in order to analyse synthetic aperture radar (SAR) images of the sea surface. One possibility is to assume a linear model for wakes, in which case detection approaches are based on transforms such as Radon and Hough. These express the bright (dark) lines as peak (trough) points in the transform domain.

In this project, ship wake detection is posed as an inverse problem, which the associated cost function has taken various forms, such as

** Generalized minimax concave (GMC) function [1,2],
** Non-convex Cauchy-based penalty function [3,4],
** Hybrid penalty function which combines the Cauchy-based penalty with a 2-D DWT based term [5].

Despite having a non-convex regularizer, we provide mathematically consistent solutions, by either

** keeping the overall cost function to be convex [1,2], OR
** setting model parameters to guarantee the convergence [3-5].

To quantify the performance of the proposed methods, various types of SAR images are used, corresponding to TerraSAR-X, COSMO-SkyMed, Sentinel-1, and ALOS2. The performance of various priors in solving the proposed inverse problem is first studied by investigating the GMC along with the L1, Lp, nuclear and total variation (TV) norms.

Algorithm

Example Results

Further information

[1] Karakuş, O., & Achim, A. (2019, May). Ship wake detection in X-band SAR images using sparse GMC regularization. In ICASSP 2019-2019 IEEE International Conference on Acoustics, Speech and Signal Processing (ICASSP) (pp. 2182-2186). IEEE.

[2] Karakuş, O., Rizaev, I., & Achim, A. (2019). Ship Wake Detection in SAR Images via Sparse Regularization. IEEE Transactions on Geoscience and Remote Sensing, 58(3), 1665-1677.

[3] Yang, T., Karakuş, O., & Achim, A. (2020, October). Detection of ship wakes in SAR imagery using Cauchy regularisation. In 2020 IEEE International Conference on Image Processing (ICIP) (pp. 3473-3477). IEEE.

[4] Karakuş, O., & Achim, A. (2020). On solving SAR imaging inverse problems using nonconvex regularization with a Cauchy-based penalty. IEEE Transactions on Geoscience and Remote Sensing.

[5] Ma, W., Achim, A., & Karakuş, O. (2020). Exploiting the Dual-Tree Complex Wavelet Transform for Ship Wake Detection in SAR Imagery. arXiv preprint arXiv:2012.06663.

Contact

Oktay Karakuş, Tianqi Yang, Wanli Ma, Igor Rizaev, Alin Achim

Code

O Karakus, and A Achim. (2020): “AssenSAR Wake Detector.”
https://doi.org/10.5523/bris.f2q4t5pqlix62sv5ntvq51yjy

Funding

This work was supported by the UK Engineering and Physical Sciences Research Council (EPSRC) under grant EP/R009260/1 (AssenSAR).

Generalized-Gaussian-Rician Model for SAR Images

Posted on by o.karakus

In this project, we present a novel statistical model, the generalized-Gaussian-Rician (GG-Rician) distribution, for the characterization of synthetic aperture radar (SAR) images. Since accurate statistical models lead to better results in applications such as target tracking, classification, or despeckling, characterizing SAR images of various scenes including urban, sea surface, or agricultural, is essential. In various SAR scenes, the illuminated area may include one (or a small number of) dominating scatterer(s), and a large number of non-dominant ones. Hence, the in-phase and quadrature components of the back-scattered SAR signal become statistically iid, but non-zero-mean random variables.

No dominating scatterer (Rayleigh case)
Hybrid case (Rician case)

This idea motivates us to utilise Rician distribution whilst modelling the SAR amplitude. Therefore, the proposed statistical model is based on the Rician distribution to model the amplitude of a complex SAR signal, the in-phase and quadrature components of which are assumed to be generalized-Gaussian distributed.

The GG-Rician statistical model is a general statistical model, which covers various important amplitude and intensity statistical models as special members.

The proposed amplitude GG-Rician model is further extended to cover the intensity SAR signals. In the experimental analysis, the GG-Rician model is investigated for amplitude and intensity SAR images of various frequency bands and scenes in comparison to state-of-the-art statistical models that include

Weibull, G0, Generalized gamma, and the lognormal distribution.

The statistical significance analysis and goodness of fit test results demonstrate the superior performance and flexibility of the proposed model for all frequency bands and scenes, and its applicability on both amplitude and intensity SAR images.

Further information

[1] Karakuş, O., Kuruoglu, E. E., & Achim, A. (2020). A Generalized Gaussian Extension to the Rician Distribution for SAR Image Modeling. arXiv preprint arXiv:2006.08300.

[2] Karakuş, O., Kuruoğlu, E. E., & Achim, A. (2020, May). Modelling sea clutter in SAR images using Laplace-Rician distribution. In ICASSP 2020-2020 IEEE International Conference on Acoustics, Speech and Signal Processing (ICASSP) (pp. 1454-1458). IEEE.

[3] Karakuş, O., Kuruoglu, E. E., & Achim, A. (2020). A Modification of Rician Distribution for SAR Image Modelling.

Contact

Oktay Karakuş, Ercan E. Kuruoglu, Alin Achim

Funding

This work was supported by the UK Engineering and Physical Sciences Research Council (EPSRC) under grant EP/R009260/1 (AssenSAR).

Cauchy Proximal Splitting (CPS)

Posted on by o.karakus

In this project, we develop a proximal splitting methodology with a non-convex penalty function based on the heavy-tailed Cauchy distribution. We first suggest a closed-form expression for calculating the proximal operator of the Cauchy prior, which then makes it applicable in generic proximal splitting algorithms. We further derive the condition required for guaranteed convergence to the global minimum in optimisation problems involving the Cauchy based penalty function. Setting the system parameters by satisfying the proposed condition ensures convergence even though the overall cost function is non-convex when minimisation is performed via a proximal splitting algorithm.

The proposed proximal splitting method based on Cauchy regularisation is evaluated by solving generic signal processing examples,
** 1D signal denoising in the frequency domain,
** Two image reconstruction tasks including de-blurring and denoising,
** Error recovery in a multiple-antenna communication system.

We experimentally verify the proposed convergence conditions for various cases, and show the effectiveness of the proposed Cauchy based non-convex penalty function over state-of-the-art penalty functions such as L1 and total variation (TV) norms.

Further information

[1] O. Karakuş, P. Mayo and A. Achim, “Convergence Guarantees for Non-Convex Optimisation With Cauchy-Based Penalties,” in IEEE Transactions on Signal Processing, vol. 68, pp. 6159-6170, 2020, doi: 10.1109/TSP.2020.3032231.

Code

O Karakus, A Achim. (2020): Cauchy Proximal Splitting (CPS).
https://doi.org/10.5523/bris.15y437loa26cr2nx8gnn3l4hzi

Contact

Oktay Karakuş, Perla Mayo, Alin Achim

Funding

This work was supported
** in part by the UK Engineering and Physical Sciences Research Council (EPSRC) under grant EP/R009260/1 (AssenSAR),
** in part by a CONACyT PhD studentship under grant 461322 to Mayo,
** in part by a Leverhulme Trust Research Fellowship to Achim (INFHER).